Quantitative Aptitude Notes: Simple Interest
INTRODUCTION:
Amount (A): The sum of
interest and principal is called Amount.
Principal (P): The sum
borrowed is called the principal.
Interest (I): Interest is
the money paid for the use of money borrowed.
Amount (A) = Principal (P) + Interest (I)
Simple Interest (S.I.): If the
interest on a sum borrowed for certain period is reckoned uniformly, then it is
called simple interest.
Let
Principal = P, Rate = R% per annum (p.a.) and Time = T years. Then
S.I = (P × T ×
R)/100
Example: A sum fetched a total simple interest of Rs. 4016.25 at
the rate of 9 p.c.p.a. in 5 years. What is the sum?
Solution:
Principal =
Rs. (100 × 4016.25)/9 × 5 = 401625/45 = Rs.8925
Note: If the rate of interest is specified
in terms of 6- monthly rate, we take time in terms of 6 months. Also, the
half-yearly rate of interest is half the annual rate. That is if the interest
is 10% per annum is to be charged six-monthly, we have to add interest in every
six month @ 5%.
Memory Based Problems based of various types
Type 1 – Basic Problems
1. Arun took a loan of Rs. 1400 with simple interest for as many
years as the rate of interest. If he paid Rs.686 as interest at the end of the
loan period, what was the rate of interest?
Solution:
Let rate =
R% Then, Time, T = R years; P = Rs.1400; SI = Rs.686
SI =
PTR/100 => 686 = 1400 x R x R/100 => R2 = 49 => R = 7
Therefore,
Rate of Interest was 7%
2. A certain sum of money amounts to Rs.1008 in 2 years and to
Rs.1164 in 3.5 years. Find the sum and rate of interest.
Solution:
S.I. for 1
½ years = Rs (1164 - 1008) = Rs 156.
S.I. for 2
years = Rs (156 x 2) / (1 ½) = Rs (156 x 2 x 2/3) = Rs 208.
Principal =
Rs (1008 - 208) = Rs 800.
Now, P =
800, T= 2 and S.I. = 208.
Rate = (100
x S.I.) / (P x T) = [ (100 x 208)/(800 x 2)]% = 13%
3. In what time will the simple interest on Rs 400 at 10% per annum
be the same as the simple interest on Rs 1000 for 4 year at 4 % per annum?
Solution:
Here , P=
Rs 1000, T= 4 yrs, R= 4 %
where, P=
Principal, T= Time, R= Rate
Since ,
Simple Interest on Rs 1000=(1000 × 4 × 4)/100 = Rs 160
now, simple
interest=Rs 160
P = Rs 400
R = 10 %
then,
T=(100 × SI)/P × R = (100 × 160)/(400 × 10) = 4 yr
4. The difference of 13% per annum and 12% of a sum in 1 year is Rs
110.Then the sum is?
Solution:
Let the sum
be 'y'
Then, [(y
x13 x 1)/100 ]- [( y x 12 x 1) / 100]=110
Since (y /
100) = Rs 110
∴ Y = Rs
11000
5. Harsha makes a fixed deposit of Rs. 20000 in Bank of India for a
period of 3 yr. If the rate of interest be 13% SI per annum charged half -
yearly, what amount will he get after 42 months?
Solution:
Give, time
= 42 months.
= (42/12)
yr = 31/2 yr
= 7 half -
yr,
rate =
13/2% half - yearly
S.I =
(20000 x 13 x 7)/(100 x 2) = Rs. 9100
∴ Amount (A)
= 20000 + 9100 = Rs. 29100
6. A moneylender finds that due to a fall in the rate of interest
from 13% to 121/2% his yearly income diminishes by Rs. 104. His capital is?
Solution:
Let capital
= Rs. P
Then, S.I1 –
S.I2 = 104.
⇒ (P x 13 x
1)/100 - (P x 25/2 x 1) /100 = 104
⇒ 13P/100 -
P/8 = 104
⇒ 26P -25P =
(104 x 200) ⇒ P = 20800
∴ Capital =
Rs. 20800
7. The difference between the interest received from two different
bank on Rs. 500 for 2 year is Rs. 2.50. The difference between their rates is?
Solution:
Let the
rates be R1% and R2%.
Then, (500
x R1 x 2)/ 100 - (500 x R2 x 2)/ 100 = 2.5
⇒ 10(R1 - R2)
= 2.5
∴ Req
difference = R1 - R2 = 0.25%
Type 2 – Money Doubling – up or Tripling
1. At what rate percent per annum will a sum of money double in 8
yr?
Solution:
Let Sum =
P, Then S.I=P
As Amount A
= 2 × P
Rate R =
(100 × SI)/(P × T) = (100 × P)/(P × 8) % = 12.5 %
Alternative Method:
RT = (n – 1) * 100; where n –
no. of times i.e. doubling or tripling
Here the
money is doubling. Therefore, n = 2;
R * 8 =
(2-1 ) * 100 => 8R = 100 => R = 100/8 = 12.5 %
2. If a certain sum is doubled in 8 yr on simple interest, in how
many years will it is four times?
Solution:
Let the sum
be Rs 'y' , so amount = 2y
Simple
interest =Rs y
Let R be
the rate of interest,
R= (100 x
S.I)/(P x T) = (100 x y) / (y x 8) = 12.5 %
now, the
needed amount = Rs 4y
since SI =
Rs (4x-x)= Rs 3y
since T=
(100 x S.I)/(P x R)
= (100 x
3y)/(y x 125)= 24 yr
3. At what rate percent per annum simple interest, will a sum of
money triple itself in 25 year?
Solution:
Let
principal amount = P
As amount
=3P, T=25 yr
∴ S.I = 3P -
P = 2P
∴ Rate R =
(100 x S.I) / (P x T) = (100 x 2P)/(P x 25)=8%
4. At the certain rate of simple interest, a certain sum doubles
itself in 10 years. It will treble itself in?
Solution:
Let
principal = P. Then, S. I = P. and Time = 10 years
∴ Required
time = [(n - 1) x t] / (m - 1)
= [(3 - 1)
x 10] / (2 - 1) = 20 years
5. In how many years will a sum of money double itself at 12% per
annum?
Solution:
Let
principal = P.
Then, S.I =
P,
Rate (R) =
12%
Time = (100
x SI) / (R x P) = (100 x P) / (P x 12) years
= 25/3
years
= 8 years 4
months
Type 3 – Installments and Investments at different rates
1. A sum of Rs. 725 is lent in the beginning of a year at a certain
rate of interest. After 8 months, a sum of Rs. 362.50 more is lent but at the
rate twice the former. At the end of the year, Rs. 33.50 is earned as interest
from both the loans. What was the original rate of interest?
Solution:
Let the sum
of Rs.725 is lent out at rate R% for 1 year
Then, at
the end of 8 months, ad additional sum of 362.50 more is lent out at rate 2R%
for remaining 4 months (1/3 year)
Total
Simple Interest = 33.50
=> (725 ×
R × 1)/100 + (362.5 × 2R × 1/3)/100 = 33.50
=> 725R/100
+ 725R/300 = 33.50 => 725R × 4/100 = 33.50 => R = 3350 × 4/725 = 3.46%
2. Mr. Mani invested an amount of Rs. 12000 at the simple interest
rate of 10% per annum and another amount at the simple interest rate of 20% per
annum. The total interest earned at the end of one year on the total amount
invested became 14% per annum. Find the total amount invested.
Solution:
P1 = Rs.
12000, R1 = 10%; P2 = ?, R2 = 20%; R = 14%
14 = (1200 ×
10 + P2 × 20)/(12000 + P2)
=> 12000 × 14 + 14P2 = 120000 + 20P2 => 6P2 =
14 × 12000 – 120000 = 48000 => P2 = 8000
3. The rate of interest for the first 2 years is 5% for the next 3
years is 8% and beyond this it is 10% per annum. If the simple interest for 8
years is Rs . 1280. What is the principal?
Solution:
r1 = 5%, r2
= 8%, r3 = 10%,
t1 = 2
years, t2 = 3 years, t3 = 8 - (2 + 3) = 3 years
∵ Principal
= (Interest x 100) / [(r1 x t1) + (r2 x t2) + (r3 x t3)]
= (1280 x
100) / (5 x 2 + 8 x 3 + 10 x 3)
=
128000/(10 + 24 + 30)
= 128000/64
=Rs. 2000
4. Pratap borrowed some money from Arun at simple interest. The
rate of interest for the first 3 years was 12% for the next 5 years was 16% and
beyond this it was 20%. If the simple interest for 11 years was more than the
money borrowed by Rs. 6080. What was the money borrowed?
Solution:
Let the sum
be P.
SI = SI for
first 3 years + SI for next 5 years + SI for next 3 years
⇒ P + 6080 =
(P x 12 x 3) / 100 + (P x 16 x 5) / 100 + (P x 20 x 3) / 100
⇒ P + 6080 =
(36P + 80P + 60P) / 100
⇒ 100 x (P +
6080) = 176P
∴ P = 608000
/ 76 = 8000
5. The annual payment of Rs. 160 in 5 yr at 5 % per annum simple
interest will discharge a debt of?
Solution:
Given,
annual payment = Rs. 160
R = 5%, T =
5 yr debt, P = ?
According
to the formula.
Annual
payment = 100*P / [100 x T + {RT (T - 1)/2}]
⇒160 = 100P
/ [5 x 100 + {(5 x 4 x 5)/2}]
⇒ 160 =
100P/550
∴ P = (550 x
160) / 100
= 55 x 16 =
Rs. 880
6. A sum was put at simple interest at a certain rate for 2 years .
Had it been put at 3% higher rate, it would have fetched Rs 300 more. The sum
is
Solution:
Let the sum
be P.
And the
original rate be y% per annum.
Then new
rate=(y+3)% per annum
According
to question, [(P × (y+3) × 2)/100]=[(P × y × 2)/100]=300
∴ [(Py +
3P)/100]=[Py/100] = 150
∴ Py+ 3P -
Py=15000
∴ 3P=15000 =>
∴ P= 5000
Thus, the
sum is Rs 5000
Type 4 - Miscellaneous
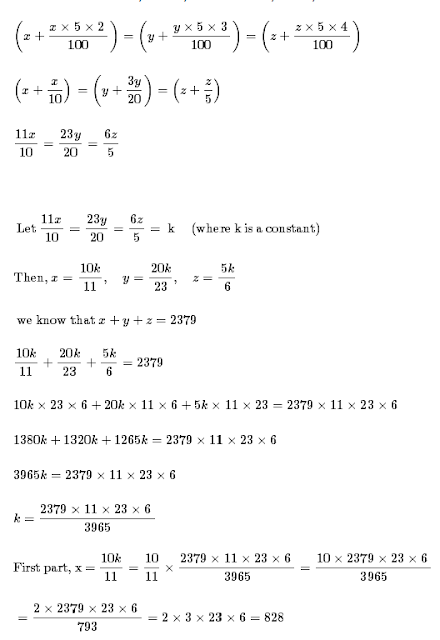
1. Divide Rs. 2379 into 3 parts so that their amount after 2,3 and
4 years respectively may be equal, the rate of interest being 5% per annum at
simple interest. The first part is
Solution:
Let the
parts be x, y and z; R = 5%
x +
interest on x for 2 years = y + interest on y for 3 years = z + interest on z
for 4 years
2. A person closer his account in an investment scheme by withdrawing
Rs. 10000. One year ago, he had withdrawn Rs. 6000. Two years ago, he had
withdrawn Rs. 5000. Three years ago, he had not withdrawn any money. How much
money had he deposited approximately at the time of opening the account 4 yr
ago, if the annual simple interest is 10%?
Solution:
Suppose the
person had deposited Rs. P at the time of opening the account.
∴ After one
year, he had
P + (P x 10
x 1)/100 = Rs. 11P/10
After two
years, he had
11P/10 +
(11P/10 x 10 x 1)/100 = Rs. 121P/100 ...(i)
After
withdrawn Rs. 5000 from Rs. 121P/100, the balance = Rs. (121P - 500000)/100
After 3 yr,
he had
(121P -
500000)/100 + [(121P - 500000)/100 x 10 x 1]/100 = 11(121P - 500000)/100 ...
(ii)
After
withdrawn Rs. 6000 from amount (ii) the balance = (1331P/1000 - 11500)
∴ After 4 yr,
he had Rs. (1331P - 5500000)/1000 + 10% of Rs. (1331P - 5500000)/1000 = Rs.
(11/10) x (1331P/1000 - 11500) ... (iii)
After
withdrawn Rs.10000 from amount (iii) the balance =0
∴ 11/10(1331P/1000
- 11500) - 10000 = 0
⇒ P =
Rs.15470
3. In 4 yr, Rs. 6000 amounts to Rs. 8000. In what time at the same
rate, will Rs. 525 amount to Rs. 700?
Solution:
Amount =
Rs. 8000, Time (T) = 4 yr; Principal (P) = ₹ 6000
Simple
interest (SI) = Amount - Principal = 8000 - 6000 = Rs. 2000
According
to the formula. S.I = (P x R x T)/100
⇒ 2000 =
(6000 x R x 4)
⇒ R = (6000
x 100)/(6000 x 4) = (25/3) %
Now, again
Amount (A) = Rs. 700
Principle
(P) = Rs. 525, Rate (R) = 25/3%
Simple
interest = A – P ⇒ 700 - 525 = Rs. 175
Using
formula, S.I = (P x R x T) / 100
⇒ 175 = [525
x (25/3) x T] / 100
⇒ T = (175 x
100 x 3) / (525 x 25) = 4 yr
4. Reena had Rs. 10000 with her, out of this money she lent some
money to Akshay for 2 yr at 15% simple interest. She lent remaining money to
Brijesh for an equal number of years at the rate of 18%. After 2 yr Reena found
that Akshay had given her 360 more as interest as compared to Brijesh. The amount
of money which Reena had lent to Brijesh must be?
Solution:
Let the
money lent to Akshay = Rs. P
Then, money
lent to Brijesh = Rs. (10000 - P) [as total amount = Rs. 10000]
S.I for
Akshay = (P x 15 x 2)/100 = 3P/10
S.I for
Brijesh = {(10000 - P) x 18 x 2}/100 = 9/25 (10000 - P)
According
to the given condition, (3P/10) - [(9/25) x (10000 - P ) = 360
[as S.I
(Akshay) – S.I (Brijesh) = 360]
⇒ (3P/10) -
3600 + 9P/25 = 360
⇒ 3P/10 +
9P/25 = 360 + 3600 = 3960
⇒ 33P/50 =
3960 ⇒ P = 3960 x
50/33 ⇒ P = 6000
∴ The amount
of money lent to Brijesh
= 10000 -
6000 = Rs. 4000
5. Rajnish invested certain sum in three different schemes P, Q and
R with the rates of interest 10% per annum, 12% per annum and 15% per annum,
respectively. If the total interest accrued in 1 yr was ₹ 3200 and the amount
invested in scheme R was 150% of the amount invested in scheme Q. what was the
amount invested in scheme Q?
Solution:
Let a, b
and c be the amount invested in schemes P, Q and R, respectively.
Then,
according to the question,
[(a x 10 x
1)/100] + [(b x 12 x 1)/100] + [(c x 15 x 1)/100] = 3200
⇒ 10a + 12b
+ 15x = 320000 ..... (i)
Now, c =
240% of b = 12b/5 .... (ii)
and c =
150% of a = 3a/2
⇒ a = 2c/3 =
(2/3 x 12/5) b = 8b/5 .....(iii)
From Eqs.
(i), (ii) and (iii), we get
16b + 12b +
36b = 320000
⇒ 64b =
320000 => ∴ b = 5000
∴ Sum
invested in scheme Q = Rs. 5000